

Mathematics-Online lexicon: Annotation to

|
[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] |

|
|
Mathematics-Online lexicon: Annotation to | ||
Mean Value Theorem | ||
| A B C D E F G H I J K L M N O P Q R S T U V W X Y Z | overview |
![\includegraphics[width=0.6\linewidth]{mittelwertsatz}](/inhalt/aussage/aussage172/img4.png)
The geometric interpretation of this identity is that the tangent at ![]() is parallel to the secant connecting the points
is parallel to the secant connecting the points ![]() and
and ![]() .
This suggests the alternative notation
.
This suggests the alternative notation
![]() for
describing the mean value theorem.
for
describing the mean value theorem.
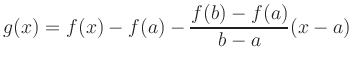
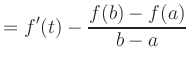 |
||
|
i.e.
| ||
| automatisch erstellt am 14. 6. 2016 |