

Mathematics-Online lexicon: Annotation to

|
[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] |

|
|
Mathematics-Online lexicon: Annotation to | ||
Principal Axis Transformation | ||
| A B C D E F G H I J K L M N O P Q R S T U V W X Y Z | overview |
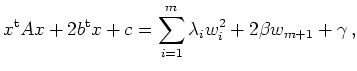
![\includegraphics[width=\moimagesize]{a_hauptachsentrafo}](/inhalt/aussage/aussage662/img4.png)
The columns of the rotation matrix ![]() contain the eigenvectors
contain the eigenvectors
![]() of
of ![]() the directions of which are called principal axes.
the directions of which are called principal axes.
| automatisch erstellt am 19. 8. 2013 |