

Mathematik-Online lexicon:

|
[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] |

|
|
Mathematik-Online lexicon: | ||
Real Fourier-Series | ||
| A B C D E F G H I J K L M N O P Q R S T U V W X Y Z | overview |
![\includegraphics[clip,width=.9\linewidth]{b_fourier_reihe_f}](/inhalt/beispiel/beispiel729/img2.png)
|
|

|
(i) Cosine coefficients:
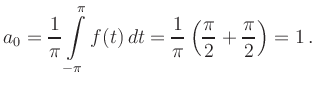
 |
(ii) Sine coefficients:
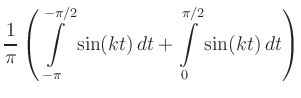 |
|||
![$\displaystyle \frac{1}{\pi} \left( \left[
-\frac{\cos(kt)}{k}\right]_{-\pi}^{-\pi/2} +\left[
-\frac{\cos(kt)}{k}\right]_0^{\pi/2} \right)$](/inhalt/beispiel/beispiel729/img11.png) |
|||
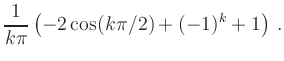 |
(iii) Fourier series of ![]() :
:
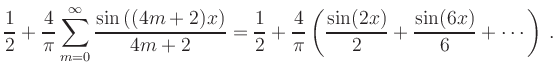
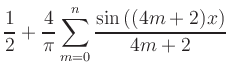
![\includegraphics[clip,width=.9\linewidth]{b_fourier_reihe_f_p1_p8}](/inhalt/beispiel/beispiel729/img28.png)
Since ![]() is not continuous, the convergence of the Fourier series is
very slow.
We notice oscillations in the vicinity of the jump discontinuities,
which is referred to as Gibb's phenomenon.
is not continuous, the convergence of the Fourier series is
very slow.
We notice oscillations in the vicinity of the jump discontinuities,
which is referred to as Gibb's phenomenon.
see also:
| automatisch erstellt am 22. 9. 2016 |