

Mathematics-Online lexicon:

|
[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] |

|
|
Mathematics-Online lexicon: | ||
Hesse Matrix | ||
| A B C D E F G H I J K L M N O P Q R S T U V W X Y Z | overview |
Let ![]() be a scalar function with continuous second partial derivatives.
The symmetric matrix
be a scalar function with continuous second partial derivatives.
The symmetric matrix
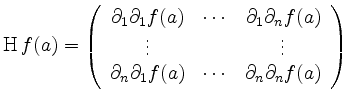
is called the Hesse matrix of ![]() at
at ![]() The Hesse matrix consists of the
second partial derivatives of
The Hesse matrix consists of the
second partial derivatives of ![]()
The expansion of ![]() in a Taylor series till partial derivatives of second
order has with the Hesse matrix the form
in a Taylor series till partial derivatives of second
order has with the Hesse matrix the form
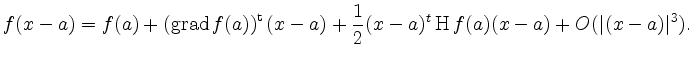
Annotation:
| automatically generated 8/ 4/2008 |