

Mathematics-Online lexicon:

|
[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] |

|
|
Mathematics-Online lexicon: | ||
Parameter Integrals and Iterated Integrals | ||
| A B C D E F G H I J K L M N O P Q R S T U V W X Y Z | overview |
Let
![]() be a continuous real scalar function.
Fix an index
be a continuous real scalar function.
Fix an index ![]() An integral of the form
An integral of the form
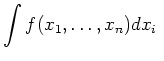 or
or
where all variables ![]() with
with ![]() are regarded as constants
is called a parameter integral (the constant variables are its parameters).
Such integrals may be seen as inverse operation to the partial differentiation
with respect to
are regarded as constants
is called a parameter integral (the constant variables are its parameters).
Such integrals may be seen as inverse operation to the partial differentiation
with respect to ![]()
An iterated integral is a sequence of parameter integrals of the following form.

It is evaluated from the inside out. Iterated integrals may analogously be defined for each ordering of the variables.
| automatically generated 6/ 1/2005 |