Mathematik-Online lexicon: Types of Critical Points

	[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff]
	Mathematik-Online lexicon:
	Types of Critical Points

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z

overview

We determine the critical points of the function

$\displaystyle f(x,y)=y(1-x^2-y^2)$

and their type.

First, we compute gradient and Hesse matrix:

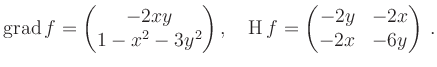
$\displaystyle \operatorname{grad}f=\begin{pmatrix}-2xy\\ 1-x^2-3y^2\end{pmatrix},\quad \operatorname{H}f=\begin{pmatrix}-2y & -2x\\ -2x & -6y\end{pmatrix}\,.$

Then, the characterization $\operatorname{grad}f=(0,0)^$ t for critical points yields

$\displaystyle xy=0 \quad\land\quad x^2=1-3y^2 \,,$

i.e.,

$\displaystyle (0,\pm 1/\sqrt{3}),\quad (\pm 1,0) \,.$

The corresponding Hesse matrices are

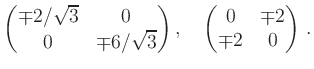
$\displaystyle \begin{pmatrix} \mp 2/\sqrt{3} & 0\\ 0 & \mp 6/\sqrt{3} \end{pmatrix},\quad \begin{pmatrix} 0 & \mp 2\\ \mp 2 & 0 \end{pmatrix}\,.$

To determine the type, we compute their determinant and trace:

$(x,y)=\left(0,\pm 1/\sqrt{3}\right)$ :

$\displaystyle \det(\operatorname{H}f)=4>0$ und $\displaystyle \quad \operatorname{trace}(\operatorname{H}f)=\mp 8/\sqrt{3}$
$\implies$ has a local minimum at $(0,-1/\sqrt{3})$ and a local maximum at $(0,1/\sqrt{3})$ .
$(x,y)=(\pm 1,0)$ :

$\displaystyle \det(\operatorname{H}f)=-4<0\,$
$\implies$ Both points are saddle points of .